Factors Which Increase The Quality Of Learning Mathematics In Primary School

The advantages of learning mathematics

From the point of view of intellectual development, the learning of mathematics helps pupils to distinguish the false from the truth, helps them to demonstrate the scientific truth, to rank their ideas, to develop logical thinking and to distinguish the essential, also, it helps them to develop their attention and to practice analysis and synthesis, to have a creative imagination, to train their logic memory, and finally, helps them to acquire the taste for knowledge.

Under aesthetic appearance, the learning of mathematics influences the personality by arousing the taste to its beauty, expressed through relations, formulas, figures and demonstrations. Also, are grown the qualities of thinking: the accuracy, the order, the clarity and elegance. Through the rigor of its feature, and not only, math forms the taste for fairness, truth and objectivity; math creates the need for verification of assumptions, math creates the need to know, to not accept anything without understanding. Math form skills of research, skills of investigation of data and problems, skills of completion of a reasoning. Math advocates for the things well made!

What else is math than an exercise of the mind preparatory for the big issues of life, of the profession or career? What else can be math than the jump over time from the stage of empirical and inefficient thinking, to heuristic thinking, that is creative? What else is math than the pattern in which we'll pour the form of our future personality? Here are just a few ideas that make us to say that there can be no knowledge which does not pass through the filter of math. It is necessary to tempt children to love math as they love their dolls and bike, or the computer. It is necessary to teach them that just deciphering the mysteries of mathematics, they can touch the limits that otherwise would seem unattainable.

Factors which influence and increase the quality of learning mathematics in primary school

Modernizing the education system, as a quality solution, is achieved on the basis of the experience accumulated in the pedagogical and practice theory. This solution is situated in the forefront of the development of pupil thinking, highlighting the qualities of thought: the flexibility and creativity. In conclusion, the realities of the dynamic world in which we live proves that mathematics remains an important pillar of the sciences and still has a fundamental role in all areas of human activities, from daily life to advanced technologies. For these reasons, mathematics must be accessible to all pupils. In recent years, math is reputed to be abstract and inaccessible, and so many pupils participate in math class with these preconceptions. Math is really abstract, but not inaccessible, and that's why teachers are those who have the role to bring it closer to new to the open minds of pupils.

So, the factors that can influence the quality of learning mathematics are (Velicu, 2016):

• aspects related to the stages of development of children’s intelligence (the classification of J. Piaget by the ages);

• aspects related to school maturity or immaturity (school maturity expresses the degree of concordance between the level of development of the child and specific school requirements);

• somatic-physiological aspects (it refers to the state of health of the pupil: health problems at birth or who appear episodic, and, of course, we can refer to school fatigue);

• aspects of the personality of the pupil (the feeling of success or failure, which is different from one person to another) (Cristea, 2000);

• aspects related to the collaboration of the teacher with pupil’s families and the community.

The pupils left behind in math can be classified into three types, by the way in which they solve their tasks:

• The dominant characteristic of the pupils from the first type is related to their personality. We refer here to those children who’s meticulously and scrupulously excessive makes them to sacrifice speed in favour of accuracy, regardless of the circumstances. They work well, but their pace slow, does not give them the opportunity to obtain a satisfactory performance. To mention that this type is specific to tired children.

• The dominant characteristic of the pupils from the second type is the presence of some slight graphic – motor difficulties. At certain tasks in which the speed and accuracy do not depend on the quality of the graphic – motor adjustments, they succeed to be effective if they don’t encounter other difficulties. But as soon as the speed or the accuracy involves some graphic skills, the work done is of poor quality.

• The dominant characteristic of the pupils from the third category consists in that the difficulties of mobilization are associated with somatic – physiological difficulties.

The factors that can increase the quality of the math lessons in Romanian primary school (Velicu, 2016):

1 ^ST Factor : the orientation of the modern didactics of mathematics in the implementation of the programs (alternative manuals and training differentiated by using some teaching strategies)

We present in the table below the differences between the traditional manuals and the modern ones, used in Romanian primary school:

We know that a strategy is a procedural scheme made up of specific actions and flexible operations, coordinated and connected to the objectives and situations through which are created the conditions of the training. A teaching strategy is the way in which the teacher helps pupils to gain access to knowledge and at the same time helps them to develop their intellectual capabilities, their skills, their feelings and emotions. Teaching strategies consist of a complex set of methods, educational techniques, means of education and various forms of organization of activities, on the basis of which the teacher develop a work plan with his pupil, in order to increase the efficiency of the didactic act.

What is a differentiated instruction? Differentiated instruction is the way of training adapted from the point of view of learning activities in terms of content, in terms of the multiple forms of the organization and of didactic methodology, to the different possibilities of the pupil, to their capacity of understanding, at the pace of the work of some groups of pupil or even of each pupil. It should be mentioned here that due to the progress of the society in a various way and not through the smoothing, the teacher does not have to erase the peculiarities of the individual, but, on the contrary, he must use them in a constructive way, he must create new material and spiritual values.

The differentiated techniques of learning mathematics are based on the gradation of tasks, according to the individual possibilities and according to the proper rhythm of development of each pupil, so the yield to be insured for the whole collective. Thus we emphasize the important role of the teacher in ensuring a climate of trust in the possibilities of each pupil, in order to be combat the complex of inferiority they probably have. So, the most important role of the teacher is to combat the trend of uniformity of the conditions of learning and to develop special conditions for those with special skills for learning (mathematics). In math class, the ways of working in this way can be:

• differentiated tasks during all activities;

• the adaptation of forms of learning;

• the adaptation of space / of the furniture according to the needs of the children;

• additional methods and procedures to meet the needs of certain children;

• additional explanations or leading questions;

• to provide individual support depending on the situations involved, depending on the interests and concerns of children;

• the pace established by the teacher (the role of the teacher is oriented to the organization of learning activities).

But to be able to train differently during the math class, the teacher must collect information about his pupil. He must register these information and disseminate it. We present below a parallel between teaching strategies applied in traditional Romanian education and the newest ones, called modern strategies:

2 ^nd Factor: the pedagogical planning of the activities, as an activity of anticipation and preparation of the instructive-educational school activities (Mocanu & Neagu, 2007; Săvulescu, 2006)

The actual planning of each lesson it is preceded by the planning of the annual and semi-annual activities. This operation involves the examination of all the material to study, taking account of the nature of knowledge, of their volume, of the purpose and of the objectives to be pursued, by the logic of the sequence of the concepts, by the skills what need to be learnt by the pupil, by the concrete possibilities of the class, the time provided in the schedule schooling, material resources existing, but and those that can be made, and last but not least, take into account the personal teaching experience of the teacher. The planning by calendar is a complex action, because it involves many elements forward-looking, what goes beyond the simple and temporary prediction.

The steps that follow a teacher in the planning of learning units can be outline like this:

See Full Size >

3 ^rd Factor : active and interactive learning of mathematics in primary school

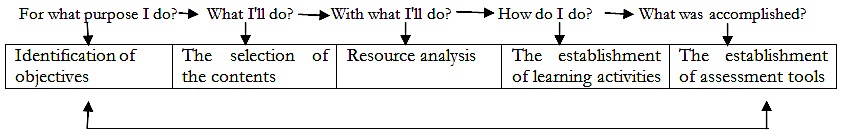
Research carried out in recent years shows that passivity in the classroom does not produce learning than in small measure. Here are a few results regarding this:

• Pupils are paying attention only 40 % of time affected of a lecture.

• Pupils retain only 70% of the contents presented in the first 10 minutes and only 20% from those presented in the last 10 minutes of the lecture.

• A study aimed at teaching focused on the speech of masterly reveals that:

• attention of pupils is decreasing with every minute that goes on during the lecture;

• lecture only fits the who learns effectively through hearing;

• the lecture promotes the learning of lower-level information;

• lecture assumes that all pupils need the same information at the same rate;

• pupils do not like to be subjected to a lecture.

Thus the yield of learning of mathematics increases when:

• pupils have a solid base of procedural knowledge (this mean ways of operating with the information);

• pupils understand the facts and phenomena into a conceptual framework;

• pupils are able to organize their knowledge so that they can be easily accessed and applied.

• pupils build their knowledge and understanding on the basis of what they already know and or think.

• pupils formulate their new knowledge by modifying and refining the concepts of their current knowledge and by adding new concepts to what they already know.

• pupils learn in a social environment in which they interact with each other, benefiting from opportunities to share and confront ideas with others.

• pupils take control of their own learning (successful pupils know when they need additional information and when they understand something, they are Mehta-cognitive, this mean they are able to monitor their ideas, thoughts and knowledge).

• pupils learn for understanding, realizing the transfer – the ability to apply knowledge in new situations.

Teachers have highlighted the beneficial effect of the interaction of the pupils during the activities in math classes. Performing tasks on groups of pupil or by didactic games, in which the pupils depend on each other, shows that:

• pupils are more involved in learning than in the front or the individual activities;

• once involved, pupils show their desire to share to the others what they are experiencing, and this leads to new connections for understanding;

• pupils come to a deep understanding when they have opportunities to explain and even teach other colleagues what they have learned.

4 ^th Factor : the interdisciplinary approach

Interdisciplinary involves the intersection ofof the different disciplinary areasby ignoring the strict limits of the disciplines. Through interdisciplinary we can address common themes to different objects of study. Through interdisciplinary appear transfers (on the horizontal) of the knowledge from one discipline to another at the methodological level and the conceptual level.

The advantages of learning at the interdisciplinary level are:

• centering of the training process on the pupil, the development of pedagogy for active and participatory work in the classroom;

• the conduct of activities on centers of interest;

• the learning is based on projects or solving problems, this mean a cooperative learning;

• the learning is lasting and with meaning, through the formation of personal competences.

The objectives of the first research were:

O₁ - the assessment of the level of knowledge and mathematical skills acquired in the earlier grades;

O₂ - identification of good practices of interdisciplinary organizing the specific contents of mathematics;

O₃ – the interdisciplinary approach to mathematics, as the determining factor of the development of thinking and creativity of children, reflected in the progress and improving their performance;

O₄ – comparison and analysis of the results obtained in the tests: initial tests, formative tests and final tests, in order to highlight the progress made by pupil;

O₅ – the valorization of research results in order to improve the didactic undertaking further in the lessons of mathematics.

The objectives of the second research were:

O₆ – the enrichment of mathematical vocabulary;

O₇ – the use of terminology specific to this discipline;

O₈ – the development of thinking and of its operations;

O₉ – the development of a strength character;

O₁₀ – the development of creativity, and of the spirit of an intellectual competition;

O₁₁ – the development of the feelings and positive attitudes;

O₁₂ – the formation of a creative habit for solving the math problems

The steps of the first research were:

• the description of the lot of subjects - analytical presentation of the experimental class via the descriptors for each pupil: first name and surname, economic level, cultural level, the number of members of the family, family type, family climate, physical development and health status, anterior school results

• synthetic presentation of the experimental class – through the percentages on the class of the descriptors listed above and the interpretation of these statistics

• synthetic presentation of the class of the control via the above descriptors and the statistic interpretation of this class

• the presentation of the methods and procedures used in the research: using the experiment as a research method and using observation as a data collection method

• carrying out the experiment: at the lot of subjects was applied interdisciplinary, and at the control class, the teacher used traditional methods

• collecting and centralizing data obtained from all the tests applied

In the second experiment we used some of active-participative methods like:

The method of conversation was used in the communication of the new knowledge, in the estimation of the knowledge acquired, in the systematization of the lessons and in fixing knowledge taught, and finally, in the activities of solving problems. The conversation consisted in a logical sequence of reproductive – cognitive questions („what is..?”, „which is .. ?”, „how..?” etc.) and productive – cognitive questions („what is the cause for..?” , „ for what purpose..?”, „ what would happen if…?”, etc.). The questions were expressed clearly, simple and concise: „ what is the question?”, „ what we know?”, „ what we need to find out?”, „ how do we find out?”, „ what operation suggests the expression in the text of the problem?” etc.

The method of observation is an activity oriented to a goal, regulated by knowledge, organized and led systematically, in a conscious and voluntary way. This method has been accompanied by other methods as well as the explanation, the demonstration, exercise and working with the manual.

The method of demonstration is an intuitive method that exploits the active character and the concrete – sensory perception of the children. The method was applied by using a wide range of materials: concrete objects for problems that can be solved by simple operations, drawings, images and graphical representations, worksheets, drawings on the blackboard and audio –visual images.

The method of creating problem situations aims for creating problem – situations that train pupils and provide them with the opportunity to capture the different relations between objects and real phenomenon, between previous knowledge and new knowledge through the solutions which they themselves find, of course, with the help of the teacher. This method involves the creation of some conflict situations, contradictory, which may result from simultaneous experience of two different realities of knowledge: the experience that holds the learner (information and skills) and the element of novelty and surprise, of the unknown (induced by a new learning activity), in front of which the old data are insufficient to solve the new task. This method involves the following moments: a trigger moment, a tensional moment and a time problem solving.

The method of the organizer chart for type of description structures request pupils to note the characteristics of some mathematical concepts (for example). The main goal pursued in the conduct and application of this method has been the formation of the personality traits, the development of spontaneity, of the initiative, of the desire for affirmation, the spirit of cooperation and courage to take decisions. The application of this method consisted in the division of the collective of the class in four groups, ensuring a balance in terms of their intellectual capabilities and taking into account the emotional relations of the pupils, followed by the communication of the tasks, solving the tasks of the group and analysis of the products of work. This method offers the possibility for updating and systematization the knowledge, but also implies certain difficulties, being necessary the intervention of the teacher.

- The method of composing problems consists in training pupil with the help of teacher (Velicu, 2016) :

- to compose problems using concrete objects, pictures or paintings;

- to compose problems according to the model previously solved;

- to compose problems using drawings or schemes;

- to compose problems which calls for the filling out of the question;

- to compose problems by completing the data when it knows the question;

- to compose problems with an indication of the mathematical operations;

- to compose problems after a previously established plan;

- to compose problems with a start gave;

- to compose problems the numerical values given by the teacher;

- to compose problems with incomplete data;

- to compose problems by adding additional data;

- to compose problems by an amendment or a correction of the content;

- to compose problems with multiple solutions or even no solutions

The conclusions of the first experiment:

Initially, at the control class the results were better than the experimental class with approximately 10%. The mistakes made by the pupils from the experimental class have been due to the inattention and lack of concentration on tasks, not because they didn't know the concepts, they have demonstrated that they have formed habits of calculation, but they are deficient in terms of the understanding and solving problems, the biggest difficulty they have encountered on solving some exercises. After applying the experiment through the application of the traditional methods at the control class and of interdisciplinary methods at the experimental class, it was observed that the number of pupil from the experimental class who have obtained a VERY GOOD qualificative increased from 50% to approximative 70 %

The conclusions of the second experiment

The advantages of using modern and active-participative strategies are :

• the climate is conducive to a fruitful cooperation between the teacher and his pupils;

• the cultivation of pupil’s responsibility for their own learning and own results;

• the appropriate setting to the teaching-learning process;

• the involvement of all pupils in the teaching-learning process;

• the development of recovery or compensatory programs, in agreement with the needs and real interests of the each pupil;

• the removal of the negative emotional states of pupils, who shuts their responsiveness and collaboration;

• the coordination of teaching to the needs of the pupil;

• the transformation of teaching lessons in an attractive and encouraging approach;

• all pupils can bring their own contribution, can be original, creative and inventive;

• the increasing of the capacity to use specific techniques in the intellectual work.

The strengths of the modern strategies are:

• the development of the motivation for learning;

• the crystallization of a style of effective learning;

• the development of the creativity;

• the formation and the development of the skills of communication;

• the formation and development of the capacity of reasoning;

• the formation and development of the emotional competencies;

• the development of self-esteem;

• the formation and the development of certain functional skills, for example some type of skills for the processing, for systematization, for restructuring and using in practice the knowledge gained;

• the development of attitudes and social behaviors;

• the cultivation of the autonomy of learning.

Weak points of the modern strategies have been observed:

• a large consumption of time;

• the generation of a group type thought;

• a superficial approach to work tasks from the pupils;

• a creation of a school climate characterized by an apparent randomness;

• an acute exacerbation of conflicts between pupil in the conditions in which the teacher does not act as a mediator;

• the risk of assimilating erroneous information, in the absence of close monitoring of the teacher;

• some difficulties in the identification and assessment of the individual progress;

• the development of a possible addiction to the group in tasks solving;

• the need of handling and numerous and various materials;

• the insufficient endowment with material from the school, which limits the use of these strategies;

• the loaded school curriculum urges the teacher to approach the traditional way, that does not consume as much time as these strategies;

• the conditioning of the transmission of rich content during the lessons in a relatively short time;

• some pupil work for others;

• the imposition of ideas or solutions from the teacher or even a fellow;

• the transmission of knowledge ready-made;

• the continues sequel of these strategies, so that they capture permanent the pupil and at the same time to create the climate of teaching;

• the risk of downtime between members of the group during the solving of the tasks.