Specifics Of The Planar Geometric Imagination And The Concept Of Its Development

Abstract

Imagination is a necessary cognitive function of everyday human activity and a prerequisite for creative thinking. We can distinguish spatial, geometric, or planar imagination but there is no clear boundary among them. In this paper, we are interested in planar geometric imagination and its development as an educational goal for student teachers at primary schools and kindergartens. Nowadays, pupils are required to develop critical thinking, be creative and be able to orientate themselves in today's world of information and images. But the same must be demanded for current student teachers training as well. There is still the concept that geometry is about drawing only. Our research covered 72 students (studying Teaching at Lower Primary Schools and Teaching at Kindergartens) of the Faculty of Education of the University of Hradec Kralove in the Czech Republic. The level of planar imagination was determined on the basis of standardized Vonkomer’s PFB planar imagination test. We measured this level at the beginning of the geometry course and at the end. In the course of geometry, students were introduced to various methods and means of teaching geometry. We also investigated whether there is a relationship between the level of plane imagination and the individual interest of students in art or playing various games in their extracurricular time. The correlation between these variables has not been demonstrated, but the positive effect of the one-semester course of geometry on the result of the planar imagination test has been proven.

Keywords: Primary educationpreschool educationmathematicsimagination

Introduction

Primarily, imagination is a cognitive process. Sternberg (Sternberg, 2002) states that imagination is an important inner process in orientation in the outside world, in decision-making and action. Therefore, the images are mental representations of those things that are not perceived by sense organs at the moment of representation. The key cognitive process involves mental representations in all sensory modalities. Visual images have all the properties of real objects in the world. They are located in a kind of mental space and these objects are mentally moved or rotated (Eysenck & Keane, 2008). Imagination is a prerequisite for creative work (Hartl, 1994).

In this paper, we will be interested in imagination in the context of mathematical abilities. The global component of imagination-related mathematical skills is the ability to think logically in the sphere of quantitative and planar relationships. The general synthesizing component that influences the type of mathematical thinking is spatial imagination (Kosc, 1972). According to Kosc (1972), one of the factors of mathematical abilities is the spatial factor. This term means the ability to perceive spatial relationships, to orient in space, or the ability to manipulate real or somehow represented material in the visual field. Furthermore, this factor involves the ability to capture purposeful and deliberate changes in the visual properties of the presented or specific objects or structures. This factor is, therefore, significantly involved in the correct solution of problems mainly in the field of geometry.

Standardized test of planar imagination

A way how to determine the level of planar imagination is to use a standardized PFB planar imagination test. This test was developed in the USA and it has been used in Czechoslovakia since 1945 as a part of a mechanical capability test. Later, the test was modified by Vonkomer (2007); however, the difficulty of the tasks has not changed. The PFB test is also considered to be a technical imagination test (Vonkomer, 2007). The test consists of 56 tasks and it has a time limit of 15 minutes. Each task shows a certain geometric shape divided into several irregular parts. The task is to compose the intended geometric shape from these parts. Mental rotation, including flipping, or, in terms of geometry, opposite isometry, is used. It is important to realize that in case of flipping, spatial imagination is necessarily involved but we work with planar patterns in mind. Some tasks may have multiple solutions, as illustrated, for example, by an easy task in Figure 01 .

See Full Size >

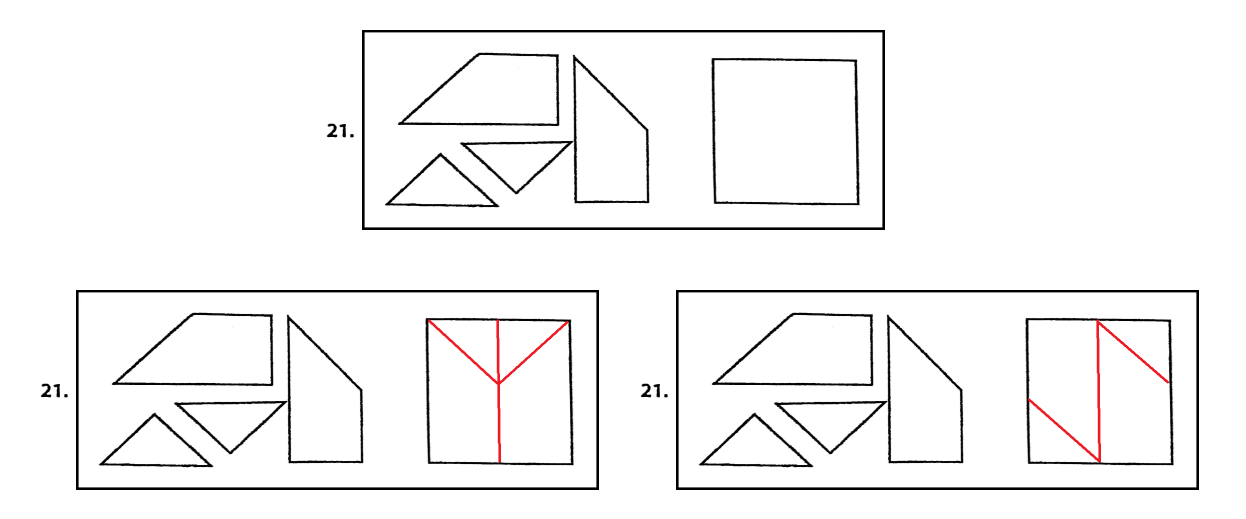
Problem Statement

We concentrate on findings focused on the level of planar imagination of primary and preschool teachers. It is obvious that, especially at primary school, the direction of education is significantly influenced by the teacher's personality.

The current curriculum of the primary stage of elementary education in the Czech Republic is directly aimed at the development of spatial imagination only within the chapter “Non-Standard Application Tasks and Problems”. But from my experience, I can say that planar and spatial imagination can be developed in any primary mathematics education curriculum, not only in geometry.

The publication called Critical Points of Elementary School Mathematics in Pupil's Tasks Solving draws attention to the low level of planar and spatial imagination. For example, in the design of constructional tasks, it has been found that emphasis is placed on the structure itself instead of analysis, which represents the moment of the idea. Sketches are taken as non-mathematical. Often, geometric shapes and tasks are given as prototypes, or "best" examples of a given category (e.g. the triangle is mainly equilateral, the parallelogram is primarily a rhomboid). The covering of the plane is not sufficiently mentioned, however, it is important for understanding the determination of the content of geometric shapes. Instead, the subject matter goes to algebraization as quickly as possible (Vondrová & Rendl, 2017).

Research Questions

We have asked these research questions:

• What is the level of planar imagination of students of primary and preschool education?

• What influence on the planar imagination of prospective teachers in primary school and kindergarten has the completion of the geometry course?

• Do these prospective teachers achieve higher scores in Vonkomer's PFB Imagination Test if they have been educated in art at high school and if they are interested in visual arts?

• Do these students achieve higher scores in Vonkomer's PFB Imagination Test if they play different games in different forms at extracurricular times?

Purpose of the Study

This study is a part of a dissertation thesis on visual arts and visual culture in teaching mathematics, where both imagination as a cognitive process and creativity are key notions. The main purpose of this study is to find out the level of planar imagination of student teachers and explore it from different directions. In addition, the findings in this study will be decisive for changing the quality of teaching geometry, algebra and arithmetic to primary school student teachers. It is equally important that the imagination in the context of the prematematical imagination of children is considered and taken into account when preparing kindergarten teachers. From ancient history, both artists and mathematicians have been researching the same natural phenomena. Since early childhood people have come to know natural and human creations through visual perception (and through smelling, touching, tasting, listening too). These experiences lead students to acquire the first mathematical concepts (Brezovnik, 2015). The article (Baker, 2013) is devoted to the positive impact of integrating art on cognitive development.

Research Methods

Findings

By processing data on prospective teachers at the University of Hradec Kralove in the Czech Republic by various statistical methods, we received answers to the above-mentioned questions, some of which were quite surprising to us.

Looking at the boxplots of the pretest and posttest results in Figure 02 , the posttest result becomes asymmetric. We can see that the students get better results in this test after a three-month geometry course. The arithmetic mean of the pretest is x ₁ = 30.5, the standard deviation of the statistical set is s ₁ = 7.4. The arithmetic mean of the posttest is x ₂ = 37.5 and the standard deviation of the statistical set is approximately s ₂ = 9.1.

See Full Size >

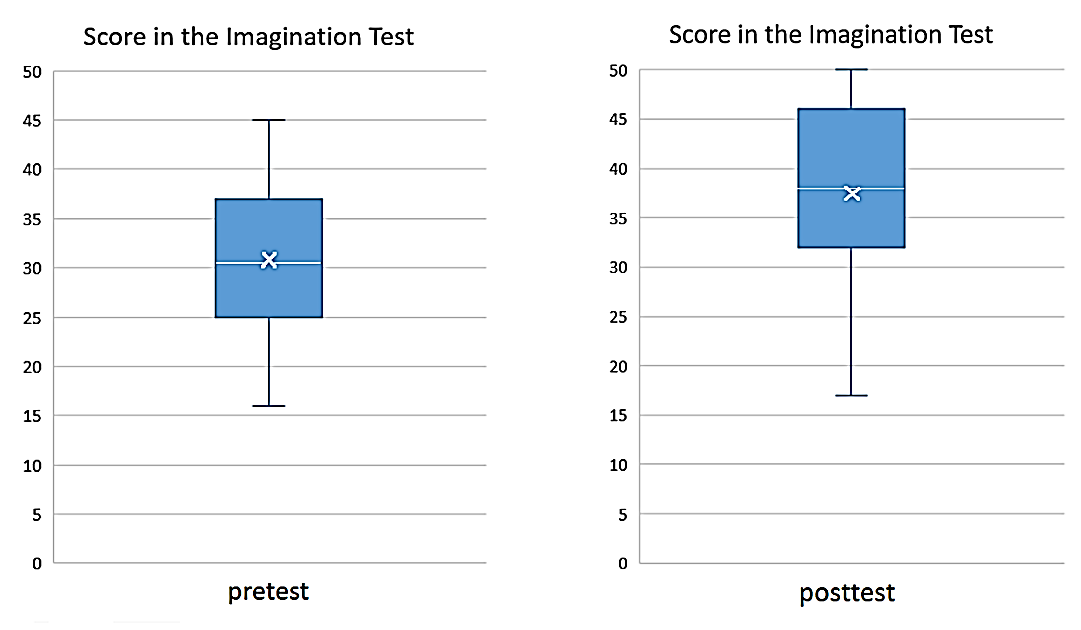
The histogram pretest and posttest deviation frequencies is shown in the following Figure 03 . Implementation of ICT via GeoGebra software could have a significant impact on improving test result. (Vallo, Rumanova, & Duris, 2014)

See Full Size >

Regarding the relationship of interest in art and the results of the imagination test, from the rough outline of the questionnaire responses, we could have expected that the effect of this factor would not be very significant. It was also confirmed by the value of the Spearmen correlation coefficient ( R _S 0.09562, critical value for n = 72 is r _0.05 = 0.232). There is no correlation between the results of the planar imagination test and the interest in art. The values of the quantity introduced by us ranged from 0 to 6. According to the responses of the student teachers of the primary school and kindergarten, they tend to incline more to music and sport.

Similarly, it has not been statistically proven whether there is a relationship between the results of the imagination test and the fact whether students play different games in their free time or not. The correlation coefficient in this case is R _S 0.22164, and thus R _S r _0.05. The values of the introduced quantity were in the range from 0 to 12.

Conclusion

Imagination, in a different sense, is important for everyday activities. Spatial imagination tests are more used in intelligence tests or assumption tests. Though, in this paper, we were interested in the planar imagination, which shifts into the spatial imagination in certain tasks. We were wondering what could be linked to the evolution of the level of imagination. We found out that there is no statistically demonstrable correlation between a high score in Vonkomer’s PFB planar imagination test and the fact whether the students have been involved in artistic work, where imagination is important. Furthermore, there was no correlation between playing games in participant’s free time and his or her results of the planar imagination test. On the other hand, the geometry courses at the Faculty of Education contributed to increase the score from this test statistically for the entire selection.

Acknowledgments

The paper has been supported by Specific Research Project of the Faculty of Education, University of Hradec Kralove, 2019, Number 10/II.

References

1. Aslan-Tutak, F., & Adams, T. L. (2015). A Study of Geometry Content Knowledge of Elementary Preservice Teachers. International Electronic Journal of Elementary Education, 7(3), 301-318.
2. Baker, D. (2013). Art Integration and Cognitive Development. Journal for Learning through the Arts, 9(1), 1-15.
3. Brezovnik, A. (2015). The benefits of fine art integration into mathematics in primary school. CEPS Journal, 5(3), 11-32.
4. Cooper, L. A., & Shepard, R. N. (1973). Chronometric studies of the rotation of mental images. New York, NY: Academic Press.
5. Eysenck, M. W., & Keane, M. T. (2008). Kognitivní psychologie [Cognitive Psychology]. Prague: Academia.
6. Hartl, J. (1994). Psychologický slovník [Psychological Dictionary]. Prague: Budka.
7. Kim, D. J., Bae, S. C., Choi, S. H., Kim, H. J., & Lim, W. (2019). Creative Character Education in Mathematics for Prospective Teachers. Sustainability, 11(6), 1730.
8. Kosc, L. (1972). Psychologia matematických schopností [Psychology of Mathematical Abilities]. Bratislava: SPN.
9. Milkova, E. (2008). Multimedia Applications and their Benefit for Teaching and Learning at Universities, WSEAS Transactions on Information Science & Applications, 5(6), 869-879.
10. Milkova, E., & Sevcikova, A. (2017). Algorithmic Thinking and Mathematical Competences Supported Via Entertaining Problems. International Journal of Education and Information Technologies, 11, 80-86.
11. Molnar, J. (2009). Rozvíjení prostorové představivosti (nejen) ve stereometrii [Development of Spatial Imagination (not only) in Stereometry]. Olomouc: Palacký University Olomouc.
12. Sternberg, R. J. (2002). Kognitivní psychologie [Cognitive Psychology]. Prague: Portál.
13. Vallo, D., Rumanova, L., & Duris, V. (2014). Spatial Imagination Development through Planar Section of Cube Buildings in Educational Process. Procedia – Social and Behavioral Sciences, 191, 2146-2151.
14. Vondrová, N., & Rendl, M. (2017). Kritická místa matematiky základní školy v řešení žáků [Critical Points of Elementary School Mathematics in Pupil's Tasks Solving]. Prague: Karolinum.
15. Vonkomer, J. (2007). PFB – Testová příručka [PFB - Test Guide]. Bratislava: Psychodiagnostika.