Information Processing As A Component Of Primary School Students’ Mathematical Competence

Abstract

In order to from one’s mathematical competence, it is already in primary school that one should be taught to understand and correctly use data from texts; to comprehend, complete, and compile tables, images; at last, to formulate statements and verify them. The following article discusses how important information processing is to the development of mathematical competence in primary school learners. Therein I regard mathematical competence as learners’ comprehension and confident use (ability to count, estimate, calculate, and solve) of the material, they have studied, both in class and after it. In this study, I analyze skill sets that contribute to information processing. I single out handling mathematical texts, seeking and presenting information in a given or self-chosen form, and handling mathematical statements. I describe how participants of the regional research (2016-2017) and the TIMSS international research test (2015) accomplished some of the tasks that required the skills under consideration. I comment on the main achievements and challenges of primary school learners and focus on the ones caused by students’ inability to process information, thus, by their lack of subject and universal skills. In the end, I suggest ways of developing information processing skills and mathematical competence at primary school. One of these ways is to encourage students to create or choose a form (table, scheme, text or other) to present mathematical information. If one understands how a model is organized, one will later be able to correctly and reasonably choose and compile information as well as improve the model.

Keywords: Information processingmathematical competenceTIMSS research

Introduction

Students learn mathematics throughout all their school years. Nevertheless, after finishing school one may fail to apply even elementary mathematical knowledge, for instance, one may find it difficult to control expenses, determine how much material they need for apartment renovation or approximately estimate the distance to or location of objects. One’s mathematical competence is generally defined by one’s ability to grasp the mathematical gist of the problem, select a necessary operations (rules and algorithms) and specific means (signs, language), use them when solving the task, test the hypothesis, and formulate the final answer. As a member of society, one needs the competence in order to catch up with different life changes. Upon developing their mathematical competence, people start to handle microcomputers, mobile information field, and multidimensional space easier. It is at primary school that children obtain the basic skills of mathematical competence, they learn to count, apply numerical methods and algorithms during classes and after them, use the language of mathematics when processing and presenting mathematical information in different forms (tables, diagrams, schemes, and other), do estimations, evaluate and regard the correctness of the answer from the mathematical perspective.

Problem Statement

Among basic interdisciplinary skills that students develop at primary school one can list such universal skills as “the application of different methods of information search …, collection, comprehension, analysis, compilation, transmission, and interpretation in compliance with communicative and cognitive purposes…” (Federal'nyj gosudarstvennyj obrazovatel'nyj standart nachal'nogo obshhego obrazovanija, 2015, p.9) In the Russian primary education information processing is regarded as a set of subject specific and universal skills (Rydze, 2017). These skills are partially developed in mathematics lessons, partially by solving task in other subjects, at last, they are gained as a result of the child’s life experience. If one considered information processing skills a component of mathematical competence, singled out their constituents, and analyzed the conditions of their successful development, one would find new ways of improving learners’ knowledge in mathematics.

Research Questions

The study analyzes separate learning skills and operations, which contribute to information processing. I specifically chose those tasks that could enable me to assess the pupil’s ability to process information. As a result, I demonstrate the learner’s achievements and challenges in information processing and suggest ways of improving mathematical competence.

Purpose of the Study

The study is aimed at singling out sets of information processing skills and describe their contribution to the mathematical competence of primary school learners.

The objectives of the study are to describe each set of information processing skills that are developed in mathematics lessons as well as to analyze students’ results in tasks that correspond to the sets.

Research Methods

The article is based on the analysis of the results of the regional research on education quality, in particular, the results of final tests in mathematics. The material was prepared by the Center for Educational Quality Assessment (under Kovaleva) of the Institute for Strategy of Education Development of the Russian Academy of Education, the Center for Educational Quality (under Soldatov) of the Academy of Public Administration of Moscow Region. The tasks for the regional tests were designed by K.A. Krasnyanskaya, M.I. Kuznetsova, and O.A. Rydze. The research also includes Russian students’ results of certain tasks taken from the TIMSS (Trends in Mathematics and Science Study) international test that was offered to primary school learners in 2015 (Mezhdunarodnoe issledovanie po ocenke kachestva matematicheskogo i estestvennonauchnogo obrazovanija, 2017). The classification of skills was supported by an experimental study on the Gymnasium in Troitsk conducted by the members of the Primary Basic Education Centre (under Vinogradova) of the Institute for Strategy of Education Development.

Findings

The study shows that information processing skills that are initially developed in mathematics lessons become later of universal character, as learners starts to use them when they handle both mathematical and non-mathematical material, at school and at home, when asked by a parent and on their own. Therefore, when describing each of the three sets of information processing skills, I will mention, first, the title and a general description of the set, second, the achievements and challenges in mastering skills at primary school, third, the prospects of using skills to develop mathematical competence and their contribution to the development of the learner’s personality.

Set One – handling mathematical texts. The issue of understanding and applying data from informational (including mathematical) texts is of particular research interest for a number of Russian and foreign scholars (Fook, 2010; Fullan & Langworthy, 2014; Lompscher, 1999; Martin & Mullis, 2013; Novoe v ocenke obrazovatel'nyh rezul'tatov, 2007; Zuckerman, Kovaleva & Kuznetsova, 2013). The researchers G.A. Zuckerman and G.S. Kovaleva admit that “the biggest gap in the comprehension of informational texts was registered in the basic reading skills of finding in the text explicit information and drawing the easiest conclusions from it” (Zuckerman, Kovaleva & Kuznetsova, 2013, p.127). Learning texts, which primary school students deal with, are typically characterised by the following: they may contain signs, special terms, descriptions of relations or interrelations, and logical links. A study designed by the Primary Basic Education Centre (under Vinogradova) in 2015-2016 proves that one of the most important universal skills a primary school learner should develop is reading for understanding. “Reading for understanding is a process of text perception, comprehension, and interpretation that results in the achievement of learning and cognitive objectives” (Vinogradova, 2016, p.145). This term is opposed to reading without understanding or reading without thinking. It is one of the purposes of successful primary school teaching to develop reading for understanding in mathematics lessons. The statement can be supported by the results of the PIRLS international research (Rossijskie shkol'niki – luchshie chitateli v mire, 2017) that have provided sufficient evidence to show that Russian primary school learners can use a text they have read to solve tasks of different complexity levels (for reproduction and production of conclusions and personal judgements).

The ability to handle a mathematical text can be characterised by the following basic skills: comprehension of the mathematical language of the text as well as adequate and correct use of text contents.

Upon analyzing how students deal with mathematical texts, one may draw the following conclusions. Russian learners find tasks (Fullan, 2014) that presuppose establishing a link between numbers written in words and in figures easy (the right answer was given by 96.6% of the participants of the TIMSS study in 2015). About 88% of learners that took part in the research chose the right response in accordance with the given set of numbers (the average world result is 64%). The most challenging tasks (under 65% of fourth graders accomplished it successfully) proved to be those that suggested that the student should read a text with specific symbols and use the symbols when they solve the problem and formulate the conclusion. M.I. Kuznetsova mentions such difficulties in her research (Kuznetsova, 2017; Zuckerman, Kovaleva & Kuznetsova, 2013), “the main challenges faced by students are connected with their inability to establish links between the knowledge they possess and the text they read, to determine whether the two coincide or not… Another challenge lies in the inaccurate interpretation of the information from the text and the inaccurate (generalized) formulation of conclusions, the final answer” (Kuznetsova, 2017, p. 500).

Primary school learners face difficulties when they are to comprehend relations and take into account all the conditions of the task. For example, they might be challenged by the following task (designed by Krasnyanskaya). “Dan has 60 stickers with superheroes. He gave 20 stickers to his brother and exchanged 10 stickers with his friend. How many stickers does Dan have now?” In 2016 72% of fourth graders from one of Russian regions accomplished the task successfully (Kovaleva et al., 2017). Around 16% of pupils misinterpreted the phrase “exchanged 10 stickers” and got the wrong answers “50 stickers” (6%), “30 stickers” (8%), and “90 stickers” (2%). I presume, if the teacher encourages students to pay attention and discuss all task conditions as well as discusses students’ mistakes that are caused by the loss of information, it will allow students to avoid such difficulties next time. Thus, the teacher may comment on the mistakes in the following way, “If you answered “50” in the task about Dan, you left fewer stickers for the poor boy because you had understood and used the word “exchanged” in the wrong way”.

Skill Set Two – information search and presentation in a given or self-chosen form. This set includes the following: understanding of the structure and contents of a table, scheme, diagram, and other models; presentation of information in a given or self-chosen form; and information reorganization for solving new learning and daily tasks.

The section Subject Results… of the primary school educational standard states that one should teach students “to deal with tables, schemes, diagrams…, and to analyze data” (Federal'nyj gosudarstvennyj obrazovatel'nyj standart nachal'nogo obshhego obrazovanija, 2015, p. 12). The Sample Program for educational institutions mentions that a primary school student learns “to plan … information search; identify the same information presented in different forms (tables and diagrams); plan elementary research, gather, and present information in tables and diagrams…” (Primernaja osnovnaja obrazovatel'naja programma nachal'nogo obshhego obrazovanija, 2015; p. 50). As students undergo their course in mathematics, they handle data from images (pictograms), blueprints, schemes, tables, and learning texts. All the models available to students are aimed at enabling them to correctly understand, generalize, classify the material, single out the main features of objects, and so on. One is supposed to master certain skills better if the way one could use them is illustrated with images.

The participants of the international comparative research TIMSS-2015 had a chance to demonstrate how they can deal with schemes (complete and blank), tables, and diagrams. When they did the task “Visit the site Find the Answer” (Martin & Mullis, 2013; Mullis, Martin, Foy, Hooper, 2016; Mullis, Martin, Ruddock, O’Sullivan, Preuschoff, 2013; Mullis, Martin, Goh, & Cotter, 2016), more than 91% of school learners showed they understood the organization of the diagram and were ready to select the column with the right answer. 74% of Russian learners managed to choose and compare columns as required. This rate is 7% higher than that of 2011.

One’s ability to understand and correctly use different models both relies on and contributes to one’s life experience. For instance, it allows one to use timetables, traffic schedules, and calendars. Thus, third graders can easily accomplish the following task: “Kate attends origami lessons on Tuesday and Thursday every week except on holidays. How many lessons will she attend in October and November (see Figure 01 ) if her autumn holidays begin on the 26th of October and end on the 5th of November?”

See Full Size >

Students may benefit from their skills of using calendars and taking into account all task conditions both in mathematics and daily life. For instance, they will later be able to solve the following task: what is the smallest and the largest number of lessons the pupil can attend if she attends them on Tuesday and Thursday? Count the number for any year required.

It is worth mentioning that Russian primary school learners find no difficulty in completing tasks where they have to present information in a table. Thus, about 94% of fourth graders correctly completed the table with the numbers they had got in the result of counting repeating objects and 94.6% of learners correctly established the relation between the information from the text and that from the diagram columns (Mezhdunarodnoe issledovanie po ocenke kachestva matematicheskogo i estestvennonauchnogo obrazovanija, 2017; Mullis, Martin, Foy, Hooper, 2016). At the same time, primary school students may find it challenging to apply their knowledge about text tasks. All pupils are aware of the condition and question that form the problem; however, a lot of them face difficulties when they have to think of additional guiding questions or make up a problem of their own with the use of the given conditions and questions. According to the research conducted at the beginning of the fifth school year (2017) in Moscow Oblast, the participants were given a condition and asked to complete it with a question so that they would design “a task for the curious. Condition. 100 g of sweetie flesh (the flesh equals half of the fruit mass) contains 45 mg of vitamin C. The daily amount of the vitamin recommended to a fifth grader is 100 mg. Nick bought a sweetie that weighed 400 g and ate it. Question______________?”. Only 56% of participants did the task correctly, they made up a question, which corresponded to the condition and the answer to which required one to use all the conditions from the task (questions starting with “Will it be enough…”, “Will he take the daily amount….?” and other).

The subject section Mathematics and Informatics of the educational standard includes “acquisition of basic computer skills” (Federal'nyj gosudarstvennyj obrazovatel'nyj standart nachal'nogo obshhego obrazovanija, 2015, p.12). The development of these skills contributes to students’ “informational education” (Abdurazakov, Suvorova, Nimatulaev & Askerov, 2017, p. 507) that presupposes that students can deal with the informational environment they are used to as well as organize data in new forms (for instance, present data in audio and media libraries, master the instructions to new gadgets, and so on).

Skill Set Three – handling mathematical statements. It includes statement formulation in the result of assignment comprehension and individual reasoning as well as statement verification (validation) based on data, solution, and supplementary sources.

The requirements of the main course in primary school mathematics (Primernaja osnovnaja obrazovatel'naja programma nachal'nogo obshhego obrazovanija, 2015) expect students to learn “to understand the simplest statements that contain link words enhancing logic (“…and…”, “if…then”, “it’s right/wrong that…”, “each”, “all”, “some”, “not”) (Primernaja osnovnaja obrazovatel'naja programma nachal'nogo obshhego obrazovanija, 2015, p. 50). Recently one has started to include more and more assignments on statement formulation and verification in mathematics textbooks. In the first and second form students learn how a sum or difference will change when one of the components of the mathematical operation is altered (for instance, they can formulate the statement “If one adds 1 to one of the addends, the sum will increase by 1”) and how they can support their reasoning with examples. In the third and fourth form the skill can be transferred to the notions of perimeter or area, for example, “If each side of a rectangle is lengthened by 1 cm, then the perimeter…”.

Primary school learners always make up a statement when they solve a problem and put down the answer. Thus, before putting down the solution to the task “In May Pete spent 100 rubles on SMS and every next month he spent 10 rubles fewer than in the previous one. How much did he spend on SMS in August?” students, first, formulate the following statement (plan): first, I will find out how much he spent in July, later the sum he spent in August, at last, I will put down the answer. If students know how to verify their statements, they can easily detect and correct the mistake (first, they should find out how much Pete spent on SMS in June). According to the English researcher J. Fook, critical reflection in the context of one’s reasoning or solution contributes to one’s motivation (Fook, 2010).

Now I would like to consider a sample task (on verification) for pupils at the beginning of their fifth school year (designed by Krasnyanskaya & Baranova). “At school fifth graders were asked to answer the question “Which of the school subjects do you like more than the others?” and choose only one option. The question was posed to 20 boys and 20 girls.

Below (see Figure 02 ) you can see the results of the poll.

See Full Size >

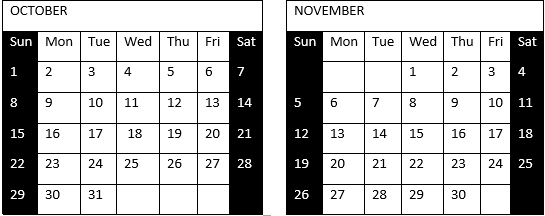
Use the diagram and say which of the following statements are true and which are false. Put ticks “V“ in the table (see Table 1 ).

The average rate of successful accomplishment is below 55% (Kovaleva & Krasnjanskaja, 2017). Conducted in the Gymnasium in Troitsk, the study of fourth-grade students’ typical challenges shows that their errors are universal and interdisciplinary rather than subject specific. Thus, one could list partial accomplishment of the task (not all questions answered), incorrect format of the answer (numbers instead of signs), and addition of new information to the diagram (columns edited and drawn by students). Furthermore, I may enumerate the following challenges connected with information processing incompetence: students cannot relate the information from the question to that in the diagram; they cannot add the values of two columns from the diagram; they cannot understand the word “only”; and they do not use markings correctly (highlight).

One of the ways to improve students’ competence is to include them in the design and selection of the form data will be presented in. If learners know how a form (table, scheme, text, and other) has been created, they will be able to repeat the process, improve it, and, if required, change it with or without the assistance of their teacher. For example, when one makes up a text, one should think of intention, plot, and the main idea. As children build their texts, they may decide, they would like to have a text problem (non-geometrical) about the number of birds that came to the bird table.

I presume, school learners will handle information (seek, select, present, and apply it) easier if they are offered tasks in thematic complexes or groups. The main feature of these so-called complex tasks would be one plot for all questions from one group. Nevertheless, the assignments may belong to different mathematical topics and even course sections. Such tasks are quite common in modern textbooks in mathematics. For instance, there is the theme In the Library in the section Mathematics Around Us in the textbook for the third grade by S.S. Minaeva et al. School learners may see an image depicting library attendance and the questions: “How many readers have come to the library today? Count the number of the visitors of the reading room from Monday to Sunday using special markings and fill in the column Total Number of Visitors per Day. Build a diagram to illustrate the table” (Minaeva, Roslova, 2016). One may also spot such tasks in TIMSS (Mullis, Martin, Ruddock, O’Sullivan, Preuschoff, 2013) and in non-Russian textbooks for primary school learners (Hoffer, Lainwand, Musser, 1991). If tasks share one plot, students can “focus on the learning task, generalize various data or data presented in different forms, plan and control the process and result of their steps, draw the conclusion, and verify it.” (Rydze, 2017).

Conclusion

After considering the results of the study, I may draw the following conclusions. First, it is possible to describe one of the components of mathematical competence, information processing, in the terms of such skills as comprehension of the mathematical language of the text, adequate and correct use of text contents; understanding of the structure and contents of a table, scheme, diagram, other models, presentation of information in a given or self-chosen form, information reorganization for solving new learning and daily tasks; statement formulation in the result of assignment comprehension and individual reasoning as well as statement verification (validation) based on data, solution, and supplementary sources. The research demonstrates that students tend to lack such information processing skills as taking into account all task conditions, accomplishing every part of the task, and generalizing information when faced with a definite problem. Meanwhile, it is worth mentioning that according to their test results, when learners finish primary school, they are fairly aware how they could use mathematical information to solve various learning and practical tasks.

Acknowledgments

The study was conducted in compliance with the State Assignment 27.7948.2017/BP

References

1. Abdurazakov, M.M., Suvorova, T.N., Nimatulaev, M.M., Askerov, A.S. (2017). Soderzhanie i sredstva obuchenija informatike v shkole v aspekte informatizacii obrazovanija. Sbornik nauchnyh trudov mezhdunarodnoj nauchno-prakticheskoj konferencii “Obrazovatel'noe prostranstvo v informacionnuju jepohu” (EEIA -2017). Moscow: FGBNU „Institut strategii razvitija obrazovanija RAO“, pp.502-509. [n Rus].
2. Federal'nyj gosudarstvennyj obrazovatel'nyj standart nachal'nogo obshhego obrazovanija. (2015). Ministry of education and science oft he Russian Federation. Moscow: Prosveshhenie. [In Rus].
3. Fook, J. (2010). Beyond reflective practice: reqorking the ‘critical’ in critical reflection. Y. Bradbury, N. Frost, S. Kulminster, M. Zukas (Eds.). London: Routledge, pp. 37-51.
4. Fullan, M. (2014). The principal: Tree keys to maximizing impact. Willey.
5. Fullan, M., Langworthy, M. A. (2014). Rich Seam: How new pedagogies are finding deep learning. London: Pearson.
6. Kovaleva, G.S. et al. (2017). Rezul'taty vypolnenija diagnosticheskih rabot po matematike, russkomu jazyku i chitatel'skoj gramotnosti uchashhimisja 5-h klassov respubliki: Tatarstan proekt «Issledovanie gotovnosti i adaptacii uchashhihsja 5-h klassov k obucheniju v osnovnoj shkole». Moscow: ISRO RAO. pp. 3-27. [In Rus].
7. Kuznecova, M.I. (2017). Diagnostika chitatel'skoj gramotnosti shkol'nikov: instrumentarij i rezul'taty. Sbornik nauchnyh trudov mezhdunarodnoj nauchno-prakticheskoj konferencii «Obrazovatel'noe prostranstvo v informacionnuju jepohu» (EEIA -2017) . S.V. Ivanova (Ed.). Moscow: FGBNU «Institut strategii razvitija obrazovanija RAO», pp. 493-502. [In Rus].
8. Lompscher, J. (1999). Motivation and activity. European Journal of Psychology of Education. N 1. M.O.
9. Martin, I.V.S. Mullis (Eds.). (2013) TIMSS and PIRLS-2011: relationships among reading, mathematics and science achievement at the fourth grade – implications for early learning. Chestnut Hill, MA: TIMSS & PIRLS International Study Center, Boston College.
10. Mezhdunarodnoe issledovanie po ocenke kachestva matematicheskogo i estestvennonauchnogo obrazovanija. (2017). Retrieved from: http://www.centeroko.ru/timss15/timss15_pub.htm (05.11.2017). [In Rus].
11. Minaeva, S.S., Roslova L.O. (2016). Matematika: 3 klass: uchebnik dlja uchashhihsja obshheobrazovatel'nyh uchrezhdenij: in 2 v. V. 2. V.A. Bulycheva (Ed.). Moscow: Rossijskij uchebnik. [In Rus].
12. Mullis, I.V.S., Martin, M.O., Foy, P., & Hooper, M. (2016). TIMSS-2015: International Results in Mathematics.
13. Mullis, I.V.S., Martin, M.O., Goh, S., & Cotter, K. (Eds.). (2016). TIMSS 2015: Encyclopedia: Education Policy and Curriculum in Mathematics and Science.
14. Mullis, I.V.S., Martin, M.O., Ruddock, G.J., O’Sullivan, C.Y., Preuschoff, C. (Eds.). (2013). TIMSS-2015: G4 Booklet 4,5. Chestnut Hill, MA: TIMSS & PIRLS International Study Center, Lynch School of Education, Boston College, pp.34-35.
15. Novoe v ocenke obrazovatel'nyh rezul'tatov: mezhdunarodnyj aspect. (2007). A. Littl, Je. Vulf. (Eds.). Moscow: Prosveshhenie. [In Rus].
16. Primernaja osnovnaja obrazovatel'naja programma nachal'nogo obshhego obrazovanija. (2015). Retreived from: http://fgosreestr.ru (26.02.2018). [In Rus].
17. Rossijskie shkol'niki – luchshie chitateli v mire! (po rezul'tatam mezhdunarodnogo issledovanija PIRLS-2016). (2017). M.: FIOKO, ISRO RAO. [in Rus.]
18. Rydze, О.А. (2017). Learners achievements in mathematical information processing at primary school. International conference "Education Environment for the Information Age" (EEIA-2017) Moscow, Russia Institute for Strategy of Education Development of the Russian Academy of Education. June 7-8, 2017. Doi: http://dx.doi.org/10.15405/epsbs.2017.08.60
19. Vinogradova, N.F. (Ed.). (2016). Universal'nye uchebnye dejstvija kak rezul'tat obuchenija v nachal'noj shkole: soderzhanie i metodika formirovanija universal'nyh uchebnyh dejstvij mladshego shkol'nika. M.: FGBNU «Institut strategii razvitija obrazovanija RAO». [In Rus].
20. Zuckerman, G.A., Kovaleva, G.S., Kuznetsova, M.I. (2013). Between PIRLS and PISA: The advancement of reading literacy in a 10–15-year-old cohort. Learning and Individual Differences, N 26, pp.64–73.